Table of Content

28 August 2023 Volume 42 Issue 8

  

Notes on the Green^,s function method

YANG Shi-jie

College Physics. 2023, 42(8):  1.  doi:10.16854/j.cnki.1000-0712.220596

Abstract ( 491 )   PDF (564KB) ( 597 )  

Related Articles | Metrics

The paper systemically describes the fundamental principle of the Green^,s function method. It demonstrates that the reversal relation holds only for the case of self-adjoint operator. The homogeneous boundary condition of the Green^,s function also comes from the self-adjointness of the differential operator while the Green integral formula does not directly apply to the non-self-adjoint operator. The paper also addresses perturbation expansion method induced by the Green^,s function, shows its relation to the popular Feynman diagram technique in physical science so as to help the student understanding the internal logics when learning advanced physics.



Several mean-value relations of a moving mass point  on elliptic trajectory under inverse squared centric force

ZHOU Guo-quan, TANG Yu-xuan, QI Ning

College Physics. 2023, 42(8):  6.  doi:10.16854/j.cnki.10000712.220359

Abstract ( 954 )   PDF (498KB) ( 1023 )  

Related Articles | Metrics

 Based on Kepler's three laws, and the conservation laws of mechanic energy and angular moment about a mass point moving on an elliptic trajectory under an inverse-squared centric force, the mean values within a period of some typical physical quantity (polar radiurs，speed, angular speed, angular momentum, rotation inertia, kinetical energy，potential energy，and so on) ，are calculated and several interesting and useful mean-value relations are found and proved. A unique mean-value point on the path of the mass point is emphasized. The Virial theorem is direct proved by means of these calculated mean values. The physical meanings, and teaching reference significance of these findings are also emphasized.



Extension and analysis of a problem on  double-conductor-sphere electrostatic induction

LI Zhao-yu

College Physics. 2023, 42(8):  11.  doi:10.16854/j.cnki.1000-0712.220435

Abstract ( 319 )   PDF (956KB) ( 461 )  

Related Articles | Metrics

A problem on the electrostatic induction of double conductor spheres and its solution are presented,and several problems are derived from it. Based on the basic idea of the mirror method, a mirror-charge construction method is designed for solving the electrostatic induction of any multiple charged conductor spheres, and the above problems are solved numerically to obtain the spatial potential and electric field in the symmetric and asymmetric cases, respectively. The charge density distribution curves on the sphere of the conductor are plotted and their physical significance is discussed.




Teaching and exploration of Fresnel half-wave zone  method assisted with VirtualLab fusion simulation 

HE Wen-qi, CUI Yue-peng, WANG Zhi-yong, LI Gui-jun

College Physics. 2023, 42(8):  15.  doi:10.16854/j.cnki.1000-0712.220424

Abstract ( 283 )   PDF (1190KB) ( 480 )  

Related Articles | Metrics

 In teaching experiment, Fresnel diffraction pattern that matches the theory cannot be frenquently and obviously observed due to the limitation of diffracting object processing precision and camera sensitivity. In this paper, VirtualLab Fusion simulation platform is used to calculate and simulate Fresnel diffraction patterns of circular aperture and stop with different sizes and different observing positions. Then the optical experiments, simulation calculations and theoretical predictions are carried out for circular apertures and stops with specific sizes respectively, and then all results are analyzed and compared with each other. It is believed that this will help to enhance teaching effectiveness.




Equipment development of metal thermal conductivity measurement with open dynamic method

ZHANG Kai-xiao, YUAN Xiao-li, XIANG Yuan-yuan, YAO Hong-bing, LEI Han, QIAN Yang-de

College Physics. 2023, 42(8):  21.  doi:10.16854/j.cnki.1000-0712.220599

Abstract ( 205 )   PDF (726KB) ( 339 )  

Related Articles | Metrics

Based on the experimental principle of Fourier thermal conductivity, in this paper an open dynamic metal thermal conductivity experimental measuring device is independently designed and developed. Through systematic analysis of the experimental measurement results and errors, the theoretical improvement model and corresponding experimental measurement methods for further improving the experimental measurement accuracy are given. The relevant experimental equipment, contents and principles have certain reference significance for college physics experiment teaching. The equipment can be used not only for physical experiment teaching and scientific research practice, but also for industrial measurement practice under open conditions.



Introduction of quantummachine learning and its application in a certain scenario

ZHU Qin-sheng, YANG Shi-lu, LIU Heng-yu, TENG Bao-hua

College Physics. 2023, 42(8):  27.  doi:10.16854/j.cnki.1000-0712.220509

Abstract ( 344 )   PDF (551KB) ( 540 )  

Related Articles | Metrics

In the past few decades, machine learning showed a profound impact on all kinds of industries. In recent years, researchers have combined quantum computing with machine learning, and proposed quantum machine learning to realize the acceleration of traditional algorithms. At present, the applications of quantum machine learning in physics, chemistry, finance, biomedicine and other fields have attracted great attention. This paper first introduces the basic concepts of quantum machine learning and the current advances. Secondly, taking hydrogen fluoride（HF） molecule as an example, we discuss the application of quantum machine learning in the calculation of ground state energy of physical systems and also demonstrate the role of quantum machine learning.



Optimal performance of endoreversible Dual cycle with non-ideal gas working fluid

CHEN Mao, GE Yan-lin, CHEN Lin-gen, XIE Zhi-hui, SHI Shuang-shuang,

College Physics. 2023, 42(8):  32.  doi:10.16854/j.cnki.1000-0712.220361

Abstract ( 142 )   PDF (785KB) ( 244 )  

Related Articles | Metrics

 Based on the finite time thermodynamic theory and the endoreversible Dual cycle model established in previous literature, this paper further considers the specific heat model of non-ideal gas working fluid, studies the cycle performance, deduces the basic optimization relationship between the cycle power and efficiency, analyzes the effects of heat transfer loss coefficient (B), cut-off ratio (ρ), freedom degree (d) of monatomic gas and the maximum temperature ratio (τ) on the cycle characteristics by numerical calculations, and compares the cycle performance differences under different specific heat models. The results show that, with the increases of τ and d, the maximum power (Pmax), the maximum efficiency (ηmax), the corresponding efficiency (ηPmax) under the maximum power condition, the corresponding compression ratios (γP,γη) under the maximum power and the maximum efficiency conditions will all increase; with the increase of B, ηmax and ηPmax will both decrease, γη will remain unchanged; with the increase of ρ, Pmax, ηmax, γP and γη will first increase and then decrease, the specific heat models have no qualitative influence but only quantitative influence on the cycle performance, the values of Pmax, ηmax, ηPmax, γP and γη under the specific heat model of the non-ideal gas condition are the minimum. The conclusions obtained in this paper have certain reference significance for the design of practical heat engines.



Research on the coupling motion mechanism of Wilberforce pendulum

LIU Rui-si, LI Xuan-qiu

College Physics. 2023, 42(8):  37.  doi:10.16854/j.cnki.1000-0712.220326

Abstract ( 293 )   PDF (920KB) ( 363 )  

Related Articles | Metrics

In this paper, an ideal model that equates Wilberforce pendulum with the coupling of spring oscillator and torsion pendulum is verified. The Lagrange dynamic equation of this model is solved. The rationality of the model is verified by designing an experiment and comparing it with the theoretical model. Then, the model is modified according to the comparison results, and a model of Wilberforce pendulum coupling motion phenomenon with closer simulation results is put forward. It is verified that there is coupling force between the up-and-down vibration and horizontal torsion of Webster pendulum, which leads to the conjecture of energy transfer.



A two-dimensional droplet shape phase transition model for  the “water spiral” phenomena

SUN Yu-wen, SHI Wei-jia, KUANG Yan-bin, ZHANG Jie, WANG Chun-mei, XIA Cheng-jie

College Physics. 2023, 42(8):  43.  doi:10.16854/j.cnki.1000-0712.220515

Abstract ( 301 )   PDF (801KB) ( 462 )  

Related Articles | Metrics

 It is well known that surface tension plays a crucial role in determining the shape and evolution of a droplet or a liquid jet. However, the specific mechanisms of some common “water spiral” phenomena remain elusive. Here, we study a two-dimensional droplet model for a liquid jet to explain the formation of water spirals by calculating the surface energy, centrifugal potential, and surface wave eigenmodes of a vibrating and rotating droplet. We find that the commonly-observed chain-like and belt-like water spirals correspond to states before and after a phase transition of the droplet^,s shape, respectively. The rationality of the current model within a certain range of parameters is justified with simple experiments.



A three-dimensional magnetic field measuring device for axial plane of  Helmholtz coil

WAN Shi-rong, GAO Wen-lei, DING Jia-wen, ZHANG Ji-cai

College Physics. 2023, 42(8):  49.  doi:10.16854/j.cnki.1000-0712.220416

Abstract ( 298 )   PDF (670KB) ( 409 )  

Related Articles | Metrics

 This paper is based on a set of Helmholtz coil axial plane magnetic field measurement device developed, combined with the PASCO experimental platform, using a two-dimensional magnetic sensor to measure the three-dimensional magnetic field component of any point on the axial plane. The magnetic field of Helmholtz coil is calculated through MATLAB programming. The comparison between the numerical calculation results and the experimental measurement data shows that the error is less than 3.0%. This experimental device has high accuracy, and the numerical calculation results can be verified by precise experimental measurement. The work in this paper further optimizes and expands the content of university physics experiments.



Visualization of similarity transformation with spin  rotating to

ZHANG Yi, LIANG Zhao-xin

College Physics. 2023, 42(8):  55.  doi:10.16854/j.cnki.1000-0712.220422

Abstract ( 223 )   PDF (420KB) ( 186 )  

Related Articles | Metrics

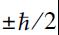
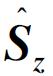
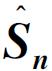
The spin of an electron is a pure quantum property without the counterpart of the classical physics. Its basic property is that the value of the spin in any direction in the spin space is. Finding a visual example to demonstrate this property of the electron spin is the key and difficult point in quantum mechanics teaching. In this work, the similarity transformation is used to rotate  to  which represents an arbitrary direction in the spin space by constructing three different rotation operators, and then the eigenvalue of the spin S^n is verified to be . 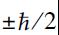Additionally, this paper shows the advantages and disadvantages of the three rotation operators in verifying the basic properties of the spin.




A new solution to the magnetic field excited by a current  carrying circular coil

WANG Yun-lang, ZHANG Tian-yi, YOU Biao, WAN Jian-guo, WANG Yin-long

College Physics. 2023, 42(8):  59.  doi:10.16854/j.cnki.1000-0712.220514

Abstract ( 307 )   PDF (777KB) ( 325 )  

Related Articles | Metrics

 In the teaching of electromagnetics in college physics, it is a very classical problem to solve the magnetic field excited by a current carrying circular coil. When dealing with this kind of problem, the traditional method is to use Biot Savart's law for vector integration The integral formula obtained by this method can not be expressed by elementary functions, and can only be approximated to the dipole form in the far field case In this paper, an innovative solution is proposed, in which the magnetic scalar potential is introduced into the space without free current to solve the Laplace equation; By using the field strength on the axis to determine the solution, the tedious process of matching the boundary conditions is avoided, and the series solution of the magnetic induction is successfully obtained Through numerical calculation, it is verified that the solution obtained by this method is consistent with the result obtained by the vector integral method, which is of great significance in electromagnetics teaching.